Exponents and radicals with interactivity

Topic outline

• Outcomes

  Collapse all Expand all
  
  In this course you will learn how to:
  
  

  1. Use exponential notation.
  2. Apply the exponent rules.
  3. Work with negative exponents.
  4. Change fractional exponents to radical form.
  5. Rationalise denominators.

  
  
  

• What are exponents?

  Just as multiplication is a short way to write repeated addition, similarly, exponents are a short way to write repeated multiplication. 

  Exponential notation is very useful to describe very large and very small numbers.

  Exponents are a powerful way to mathematically describe rapid increases or decreases in growth. We have seen an explosion of growth in technology, which can best be described using exponents. 

  Watch the next video to see how rapidly technology has grown. 

       
  

  • Mark as done

    Using exponential form

    Writing repeated multiplication in exponential form makes it easier to simplify long expressions. The next video explains how to use exponential notation.  

    
    

    
    

    
    

  • Mark as done

    Parts of a power

  • Mark as done

    
    

    
    

  • Mark as done

    ACTIVITY: Use exponential form

    
    

    
    

    
    

• Exponent rules

  The exponent rules make it simpler to work with exponents. Watch the next video for a recap of the rules of exponents and make sure to answer the questions in the video.

  
  

  
  

  • Mark as done

    Activity: Using the exponent rules

    
    

    
    

  • Mark as done

    Exponential identities and negative exponents

    You have mainly used positive exponents but exponents can be negative or even zero. When exponents are negative or zero we use special identities as discussed in the next video.  
    

  • Mark as done

    Activity: Working with negative exponents

    
    

    
    

    
    

• Fractional exponents and radicals

  A fractional exponent is the same as finding some root of a number.   We generalise this as: \({x }^{\frac{1}{n}}= \sqrt[x]{n}; n\in N\ , x\in R\).

  The root symbol has a special name, it is called a radical.  Each part of a radical has its own name, as shown below.

  

  Rewriting fractional exponents as radicals

  To change a fractional exponent to a radical, rewrite the number in the numerator of the exponent as the exponent of the radicand, and the number in the denominator of the exponent as the root as shown in the image below. 
  

  
  

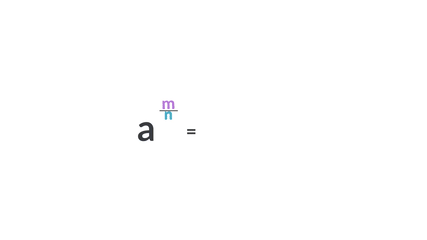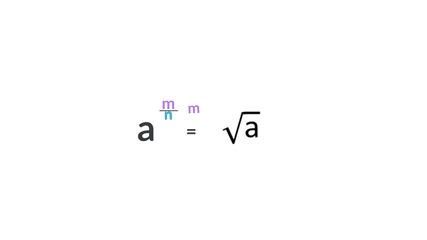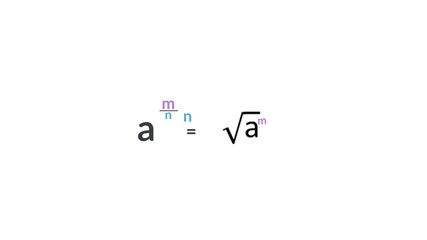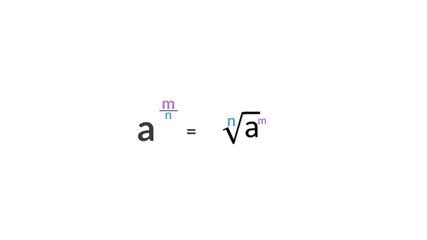
  Test your skills of changing from fractional exponents to radicals by trying the next activity.

  • Mark as done

    Activity: Rewriting rational exponents

    
    

    
    

    
    

  • Mark as done

    Rationalising denominators

    Surds are roots that cannot be reduced to a whole number or fraction. When a surd is in the denominator of a fraction we need to remove the surd to make the fraction simpler to work with. 

    The process we use to remove a surd from the denominator is called rationalising the denominator. This concept is shown in the next video.
    

    
    

    
    

    
    

  • 

    Licence & Attribution Page

    Mark as done